Estimating the Laplace-Beltrami Operator by Restricting 3D Functions

Symposium on Geometry Processing, July 2009

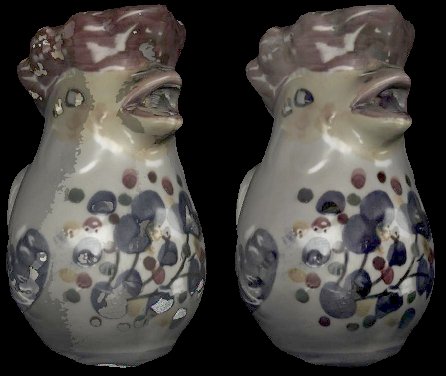
Ming Chuang, Linjie Luo, Benedict J. Brown,
Szymon Rusinkiewicz, Michael Kazhdan

Reconstructing colored surfaces from 3D scans: The texture obtained by pulling color values from the closest scans is shown on the left, while taking color gradients from the closest scans and solving the Poisson equation gives the seamless result on the right.

We present a novel approach for computing and solving the Poisson equation over the surface of a mesh. As in previous approaches, we define the Laplace-Beltrami operator by considering the derivatives of functions defined on the mesh. However, in this work, we explore a choice of functions that is decoupled from the tessellation. Specifically, we use basis functions (second-order tensor-product B-splines) defined over 3D space, and then restrict them to the surface. We show that in addition to being invariant to mesh topology, this definition of the Laplace-Beltrami operator allows a natural multiresolution structure on the function space that is independent of the mesh structure, enabling the use of a simple multigrid implementation for solving the Poisson equation.

PDF file

Ming Chuang, Linjie Luo, Benedict J. Brown, Szymon Rusinkiewicz, and Michael Kazhdan.
"Estimating the Laplace-Beltrami Operator by Restricting 3D Functions."
Symposium on Geometry Processing, July 2009.

@article{Chuang:2009:ETL,
   author = "Ming Chuang and Linjie Luo and Benedict J. Brown and Szymon
      Rusinkiewicz and Michael Kazhdan",
   title = "Estimating the {Laplace}-{Beltrami} Operator by Restricting {3D}
      Functions",
   journal = "Symposium on Geometry Processing",
   year = "2009",
   month = jul
}